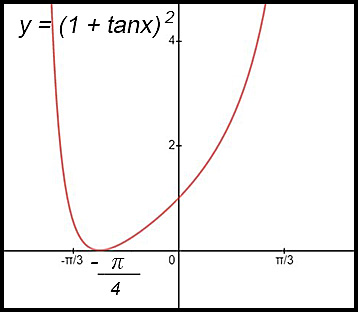
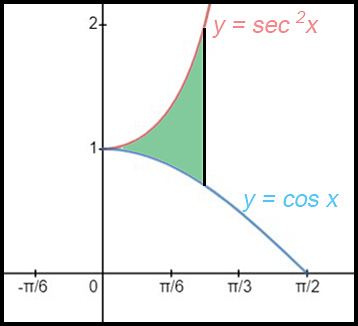
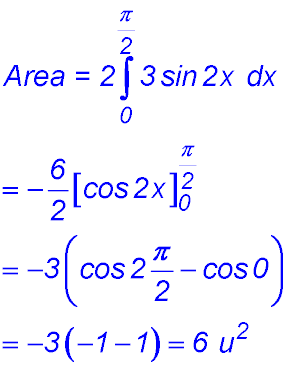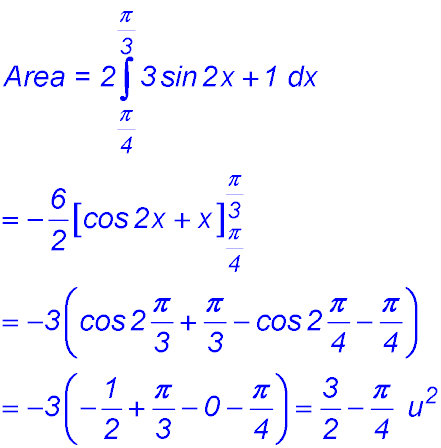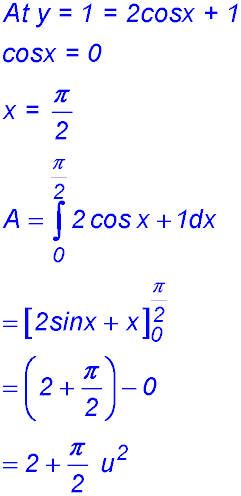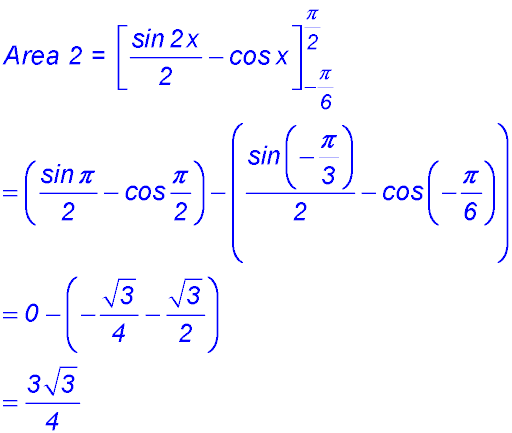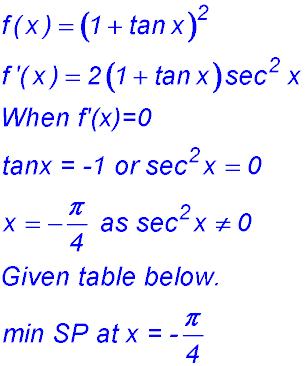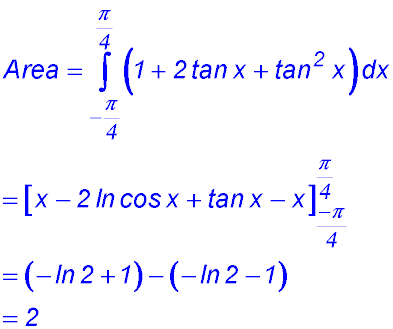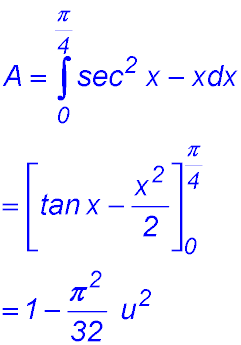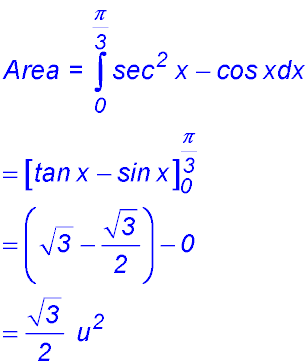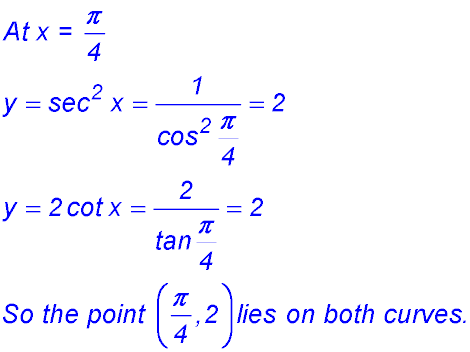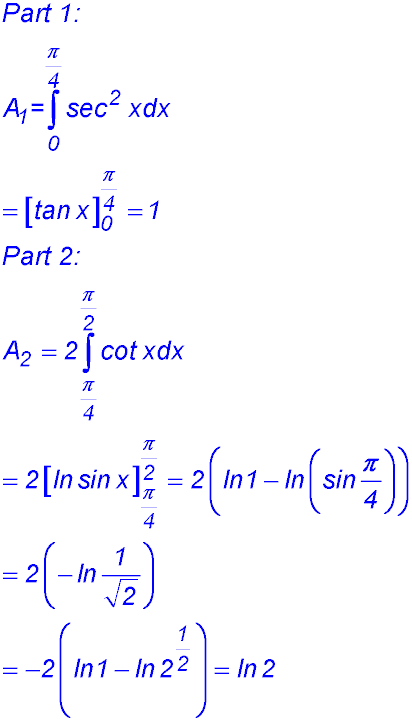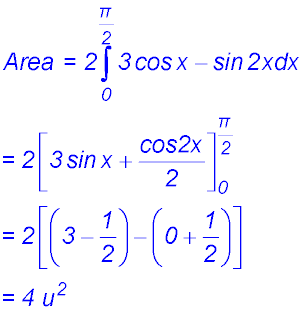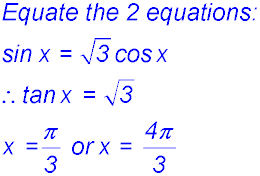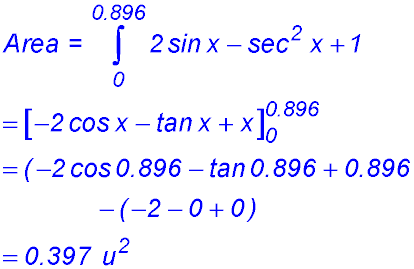Where the techniques of Maths
are explained in simple terms.
Trigonometric functions - Integration - Applications finding areas.
Test Yourself 1 - Solutions.
- Algebra & Number
- Calculus
- Financial Maths
- Functions & Quadratics
- Geometry
- Measurement
- Networks & Graphs
- Probability & Statistics
- Trigonometry
- Maths & beyond
- Index
Solve the following problems as indicated:
| Area - 1 curve |
1. 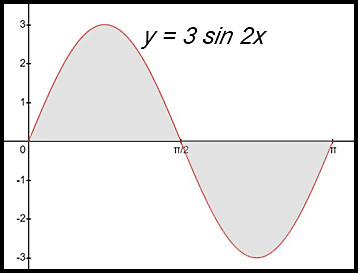
The curve is symmetrical about y = π/2. So the area between 0 and π/2 can be determined and then doubled.
|
2. (i) 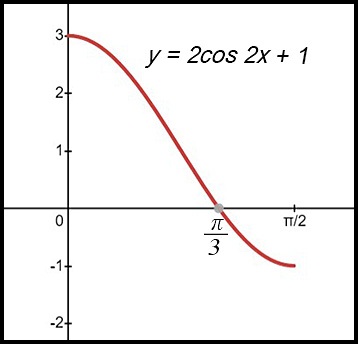
(ii) When y = 1:
∴ There are two shapes: 1. a rectangle of height 1 and length π/4. 2. the area under the curve between π/4 and π/3. For the 2nd area:
|
||||||||
3. 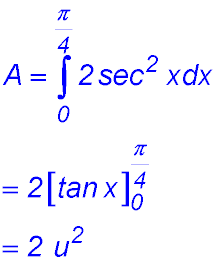 |
4. (iii) |
|||||||||
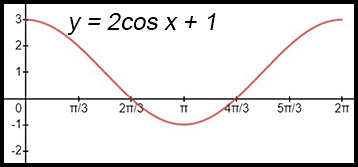
4. f(x) = 2cos x + 1,
|
||||||||||
5. (i) 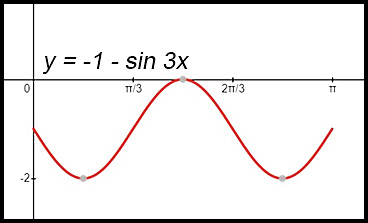
|
(ii) 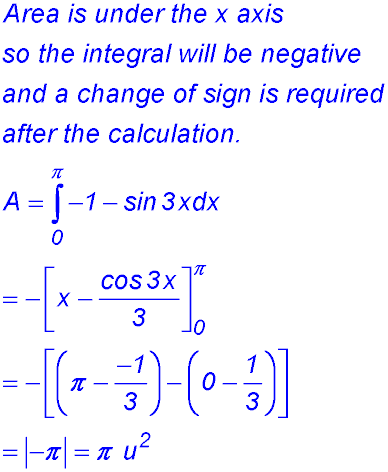 |
|||||||||
6. 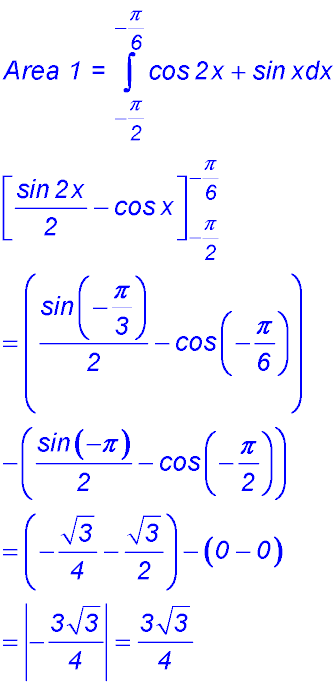 |
|
|||||||||
7. (i)
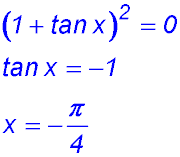
(ii)
|
(iii) (iv) |
|||||||||
8. 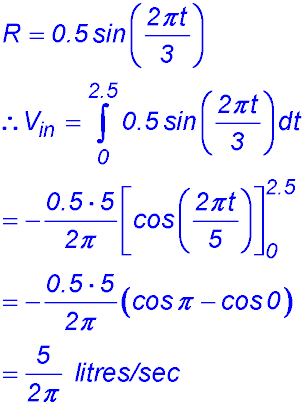 |
||||||||||
| Area - 2 curves with no point of intersection. |
9. (i) 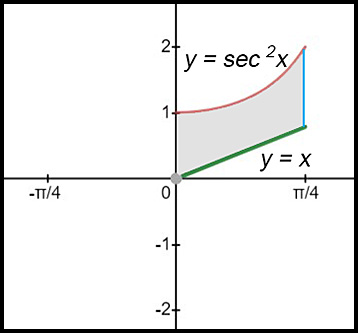
(ii) |
10. (i)
(ii) |
||||||||
| Area - 2 curves with one point of intersection |
11. 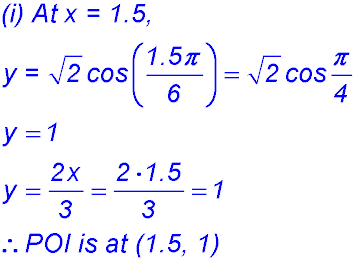 |
(ii) 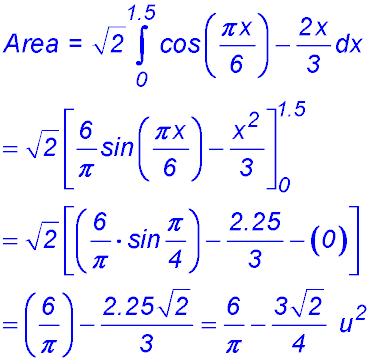 |
||||||||
12. 
(i)
(ii) |
(iii) There are two parts to integrate -
∴ Area = 1 + ln 2 |
|||||||||
13. (i) 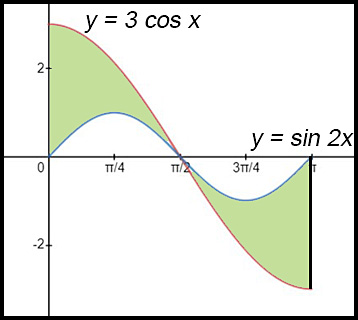 |
(ii) The two curves are symmetrical about x = π/2. So areas are doubled to obtain total areas from
|
|||||||||
14. (i) 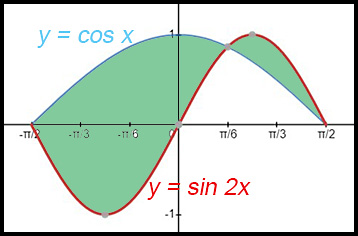 |
(ii) 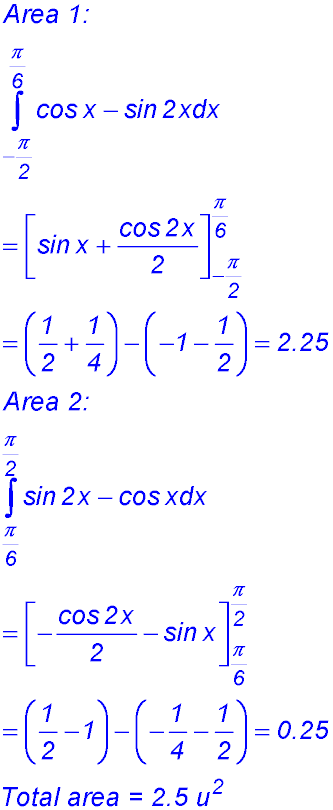 |
|||||||||
| Area - 2 curves with two points of intersection |
15. 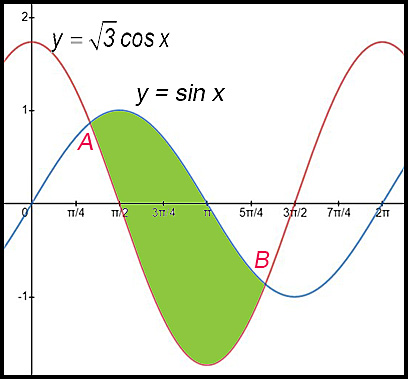
(i) |
(ii) 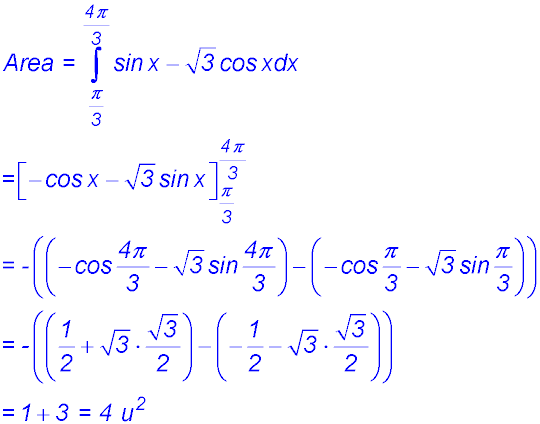 |
||||||||
16. (i) 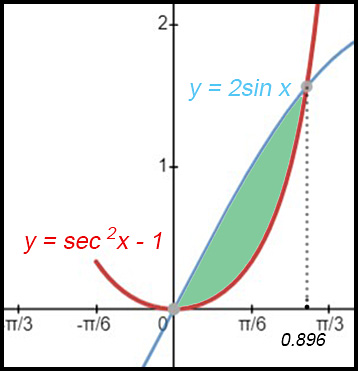
x = 0, y = 2sin x = 2 sin 0 = 0
x = 0.896, y = 2sin x = 2 sin 0.896 = 1.562
Hence the curves intersect at x = 0 and |
(ii) |